Experimental quadruple-tank network decentralized control
Updated:
Tags: control, example, experimental, ltv, mpc, nonlinear, one-step
Experimental application of decentralized control to the quadruple-tank network, using the one-step method.
This example corresponds to the experimental implementation of the numeric example “Decentralized control of nonlinear quadruple-tank network”.
The setup
This experimental example is performed on the Reproducible Low-cost Flexible Quadruple-Tank Process Experimental Setup for Control Educators, Practitioners, and Researchers proposed in [1]. This setup is very easily reproducible, costs under 650€, and takes roughly 4 hours to assemble. The CAD models, technical drawings, wiring schematics, and software are open-source and available here. A CAD model of the setup, as well as a physical implementation, can be seen below.


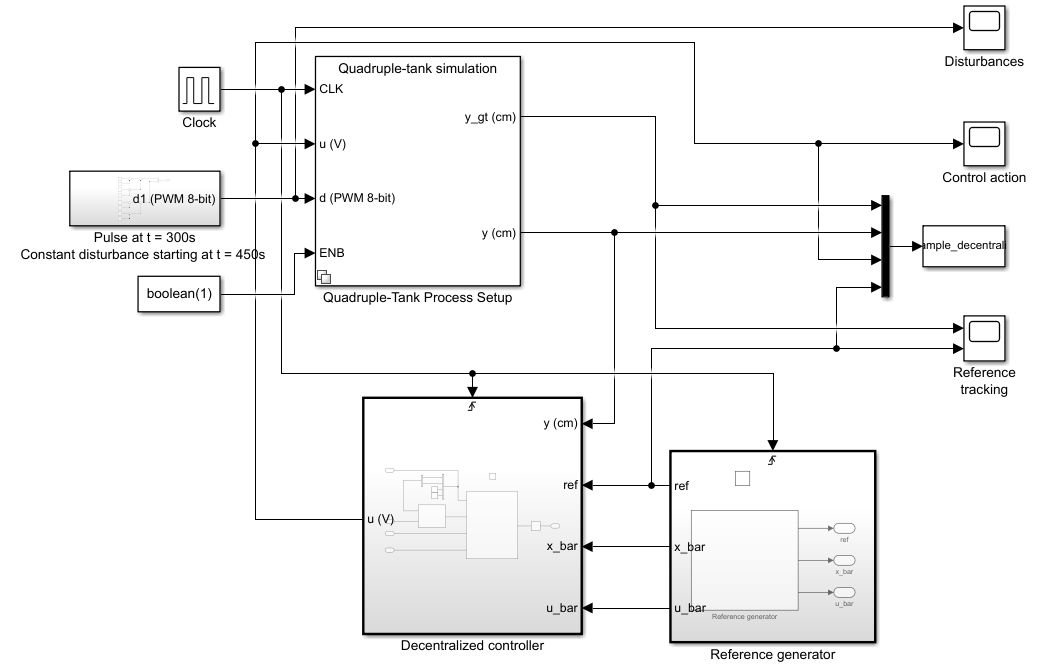
A user-friendly dedicated MATLAB/Simulink interface is openly available as well. It allows for a seamless shift between a numeric simulation and the interface with the experimental plant. The simulink model design for this example is shown below. It is available here.
Experiment
In this example, the decentralized tracker put forward in [2] for linear time-varying systems is experimentally applied to the quadruple-tank process. To employ this method, the process dynamics are approximated by an LTV system, linearizing and discretizing its dynamics about successive equilibrium points. Impulsive and constant disturbances are introduced, starting at \(t = 300 \mathrm{s}\) until the end of the simulation. A recording of the experimental simulation is shown below.
Results
The experimental results, which are analysed carefully in [1], are shown below.


The numeric simulation results are shown below.

